3 Methodology
The methodology required to answer the research questions on the value of the publicly provided trees (ecosystems) in Kamloops, their annual ecosystem services, the factor that influence the value of the trees, their spatial distribution, and finding gaps will require a multifaceted approach. Initially some descriptive statistics will be provided on the type of trees, their characteristics, and their valuation in the city and by zones. This will be followed by a multiple regression model, with data processing and statistical analysis performed in Stata. It aims to establish a statistical relationship between the appraised value of urban trees (the dependent variable) and their physical characteristics and location-specific factors (the independent variables). Using this model, we can estimate the impact of factors like a tree’s diameter, height, and geographic zone on its overall appraised value. The study area is the city of Kamloops, which is situated in British Columbia’s semi-arid interior. The city’s urban and suburban areas will be chosen according to variables like the density of trees, land use, and socioeconomic diversity. We will designate zones or neighborhoods for a more thorough and representative evaluation.
The data for this study were obtained from the Kamloops Urban Forestry Management System (UFMS), an existing city database that contained about 19,011 trees. The inventory needed to meet certain requirements to be included in this study.
- include every tree under the public domain.
- include information about each tree’s species, total height, breast height diameter (DBH), spread, space growing, tree condition, year planted, common full name and city zone.
The initial dataset of 19,011 tree records downloaded on November 12, 2024, from [data source]. To ensure data accuracy and reliability, we conducted a series of data cleaning steps. First, we removed observations where tree appraisal values were either zero or missing, reducing the dataset to 16,716 trees. Next, trees with diameter at breast height (DBH) values that were zero or missing were excluded, leaving 16,624 observations. We then removed trees with height values that were either zero or missing, resulting in 16,608 trees. Additional exclusions were made for records identified as stumps, planting or removal sites, and other deleted observations, bringing the total to 16,160 trees. Finally, we filtered out trees classified as privately owned, shared ownership, or of unknown ownership, retaining a final sample of 15,200 publicly owned trees located in Kamloops. In the case where age of the tree is controlled observations drop to 4,010. See Table X.
| Removal of observations | Original dataset | Amount removed | Number of Trees remaining |
| 19,011 | |||
| Removed zero/missing appraisal values | 2,295 | 16,716 | |
| Removed zero/missing DBH values | 92 | 16,624 | |
| Removed zero/missing height values | 16 | 16,608 | |
| Removed stumps, planting/removal sites, and deleted for site type | 448 | 16,160 | |
| Removed private, shared, and unknown ownership | 960 | 15,200 | |
| Trees publicly owned to value | 15,200 |
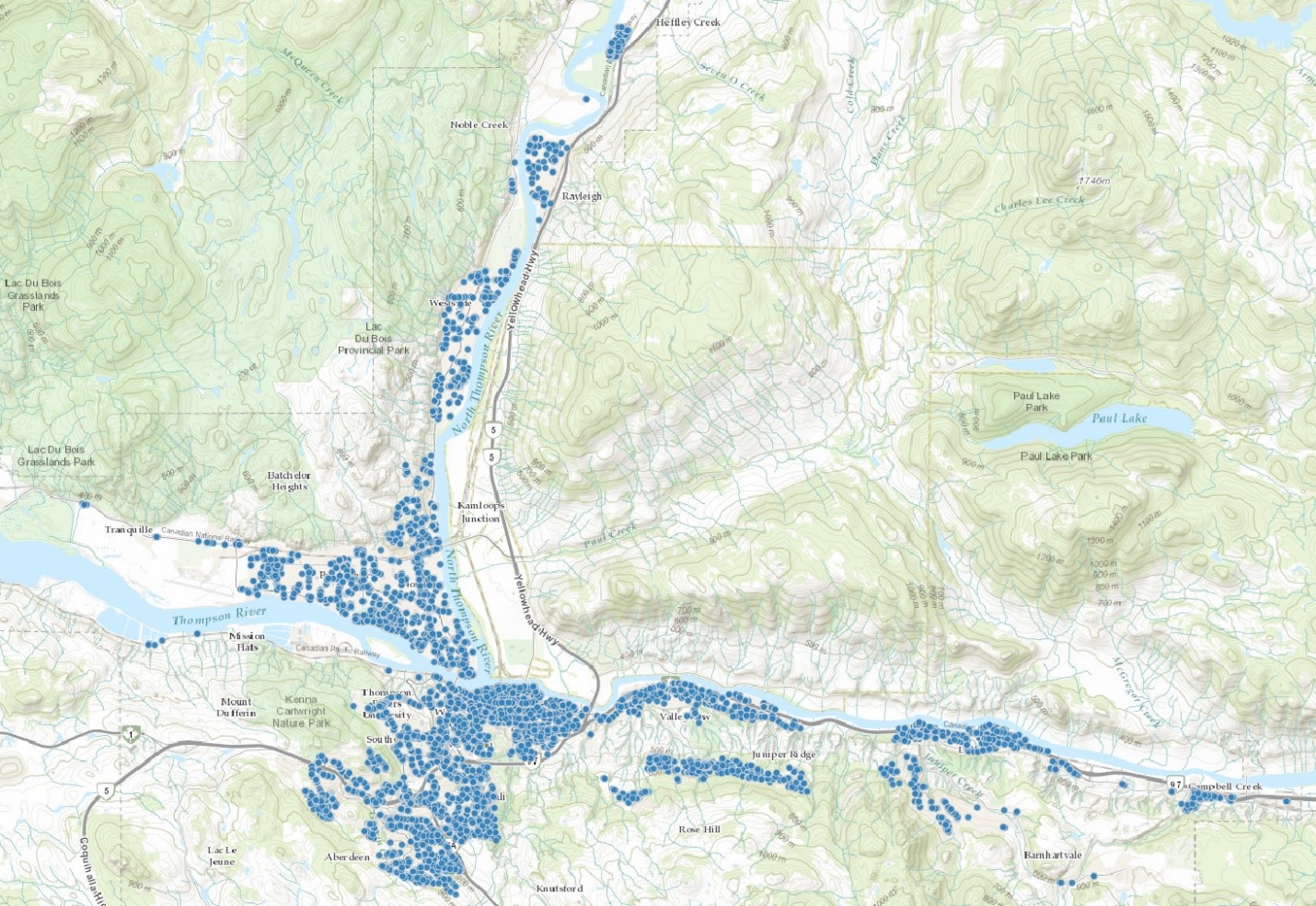
The final selection comprised 15,200 publicly owned trees in total, including the Crabapple spp. comprising over 1300 trees (7.6% of the total trees), the Green Ash comprising 943 trees (5.5%), the Douglas fir with over 500 trees (2.9%), the Chokecherry Schubert with 292 trees (1.7%), and many other species. Fig 1 shows the distribution of the public urban trees in Kamloops.
Cost Approach
The cost approach to tree valuation mainly focuses on the replacement cost method, which estimates the expense required to replace a tree with another of similar size, species, and utility. This method is widely used because it provides a clear, easily understandable monetary value that can be readily communicated to stakeholders. According to McPherson (2007), the cost-based approach, particularly the Council of Tree and Landscape Appraisers (CTLA) trunk formula method, is a key tool in estimating tree value. This method accounts for factors like tree size, species, and condition—all essential in determining an accurate replacement cost.
This approach applies two main formulas based on the tree’s size:
- Replacement Cost Method: For smaller trees that can be replaced directly from nursery stock.
- Trunk Formula Method: For larger, mature trees that are too big to be replaced in kind.
Trunk Formula Method
Both methods follow a similar formula to calculate a tree’s value: the local cost of purchasing and planting a similar tree and the specific condition and location of the tree being appraised.
The “Basic Value” of a tree is determined by several factors that reflect its overall worth:
Basic Value = Replacement Cost + (Basic Price x [TA(A) – TA(R)] x Species)
The basic value is composed of the replacement cost and its growth assessment ((Basic Price x [TA(A) – TA(R)] x Species) since the replacement may not be as mature as the one it replaces.
The replacement covers the total cost of replacing the tree, including expenses like the purchase, planting, and transportation of a similar tree if it were to be lost or damaged. It is used to assess smaller tree when the replacement is of similar type and size. For larger trees relative to the replacement the valuation requires the second term which estimates the value of the tree for its current size relative to the replacement tree which latter is of significantly smaller in size. Using the tree’s diameter at breast height (DBH), the difference between the actual and a potential replacement tree is (TA(A) – TA(R)) reflecting the loss of value of the mature tree relative to the replacement tree. This is multiplied by the basic price to get the value of this difference in the trees. The basic price is a standard rate applied based on the tree’s size, such as per inch of trunk diameter or height, with rates varying based on location, species availability, and market conditions. Finally, the value is adjusted based on the tree species’ characteristics and desirability, like longevity, beauty, rarity, or ecological value.
Then the general formula for calculating the tree’s appraised value is:
Appraised Value = Basic Value x Condition x Location
To get the final appraisal value, the basic value is multiplied by the condition of the tree and its location. The condition of a tree refers to the tree’s health and structural soundness are rated, with perfect health at 100% value. Any health issues, like disease or structural damage, reduce this rating and, therefore, the tree’s value. The tree’s placement (location) affects its value based on its benefits, like ecological contributions, visibility, and social or aesthetic value. Trees in high-impact areas, such as those reducing stormwater or enhancing curb appeal, are often valued higher. On the other hand, trees near buildings or power lines may be valued lower due to associated maintenance costs or risks.
This valuation method, guided by standards from organizations like the Council of Tree and Landscape Appraisers (CTLA) and the International Society of Arboriculture (ISA), is essential in urban planning and legal contexts where compensation for tree damage or loss may be needed. In addition to the Trunk Formula Technique (TFT) used in the CTLA method, several other methods are available for tree valuation such as:
- Helliwell System: This is a UK-based expert method that assigns scores to tree attributes (e.g., size, and life expectancy) and converts them to monetary value.
- STEM (Standard Tree Evaluation Method): A New Zealand method that adds factors together, resulting in more consistent appraisals among different evaluators.
- Norma Granada Method: A Spanish method that often generates higher values by applying unexplained “value factors.”
- Burnley Method: Developed in Australia, this method calculates tree volume and multiplies by cost per cubic meter.
- CAVAT (Capital Asset Value for Amenity Trees): Used in the UK with both detailed and quick valuation methods.
- i-Tree Suite of Tools: Focuses on estimating ecosystem services provided by trees.
- Benefit-Based Valuation: Projects the net present value (NPV) of future tree benefits using growth data.
- Income Approach: Values trees based on potential income, typically for fruit-bearing species.
- Sales Comparison Approach: Rarely used, this method values trees by comparing them to similar trees sold.
Regression Analysis
The dependent variable is the tree’s appraised value, expressed as an estimated monetary value based on social, ecological, and structural benefits. The independent variables include height (lnHeight), DBH (lnDBH), and dummy variables that represent each zone (D9RA, D8BA, etc.), in addition to other potential economic and environmental factors. The tree value (lnA) is estimated using a log-linear regression model to capture the relationships between tree size, species, zone characteristics, and appraised value. The general model is specified as follows:
InA = b0 + b1 InDBH + b2 InHeight + Sum of biDi + e

